Deal or No Deal: Gambling With Math!
I’ve caught the game show Deal or No Deal twice over the last couple weeks and it’s driving me crazy! It’s a mathematical game but the contestants don’t play it mathematically. It’s getting to the point where I can’t watch it anymore!

How the Game Works
If you haven’t seen the show, it’s a pretty simple concept. At the beginning of the game the contestant selects a briefcase containing an unknown sum of money. Throughout the game a “banker” offers the contestant a flat sum to walk away from the game (Deal) or give up the money for a chance to open their own case (No Deal).
During each round the number of cases to open changes as does the offer from the bank. The smallest amount is $0.01 and the largest amount is $1 million.
Decision Making
First I watched an episode where the player had 2 cases remaining with the following dollar amounts:
- $0.01
- $10,000
The offer from the bank was $5,500. What would you do? Unfortunately she didn’t take the offer, opened her case and was the first player to leave the show with one penny. Would it change your opinion if earlier she had already given up many higher offers, the highest at $207,000?
I also caught a variation of the game show on Oprah. The two final cases contained these amounts:
- $5,000
- $75,000
The offer from the bank was $50,000. Oprah chose to open the case…. and guess what it contained? Of course it only had the lower amount in it.
Expected Outcome
This expected outcome can easily be calculated in this game. The formula for expected outcome is:
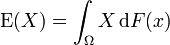
See the mathematical definition for more information.
A simple example from wikipedia is the expected value when you roll a die:
![\begin{align} \operatorname{E}(X)& = 1 \cdot \frac{1}{6} + 2 \cdot \frac{1}{6} + 3 \cdot \frac{1}{6} + 4 \cdot \frac{1}{6} + 5 \cdot \frac{1}{6} + 6 \cdot \frac{1}{6}\\[6pt] & = \frac{1 + 2 + 3 + 4 + 5 + 6}{6} = 3.5, \end{align}](http://upload.wikimedia.org/math/5/4/f/54f12b1f08853ef18606aa5a1ffd7ee6.png)
In the examples above, the expected outcome for $0.01 and $10,000 is $5,000.005. Of course there isn’t a half penny, but the offer of $5,500 is almost $500 above the expected outcome.
In the second example, the expected outcome for $5,000 and $75,000 is $40,000. The offer of $50,000 was $10,000 above the expected outcome!
What I Would Do
It pains me to watch this show. In both cases I would take the deal. In general I don’t have much risk aversion, but this type of game is all math to me. If the offer from the bank is more than the expected outcome, I will walk. I have discussed it with various family members and found some opinions are very different from mine.
I consider the money in each round already mine. In the first example, if you came up to me on the street and asked me to bet $5,500 to win either a penny or $10,000, I would think you are nuts! My money has no business participating in that kind of expected loss.
Other Considerations
Of course, it’s really easy for me to sit at home yelling at the t.v. Under the lights, with adrenaline pumping, and playing for real, I might make a different decision. I really can’t say for sure. Not to mention the family members you bring along that try to convince you which way to go.
The amount of money also has some bearing on whether following a mathematical equation makes sense. In the first example people might be more willing to risk $5,500 than they would $40,000. And Oprah has a lot of money, so her threshold is probably much higher than other people.
Action Plan
I’d like to think that I know I would adhere to mathematical principles in a mathematical game. It’s probably also the same reason I wouldn’t ever be invited to be on the show, because they would know exactly what I would do in any given situation.
This isn’t to say that I don’t gamble, I do… more about that some other day!
Deal or No Deal?





Nice blog. I share your anguish. My sister was consistently amazed when I would pretty accurately guess the bank’s offer using the methods you described. It’s pretty simple math.
I bet if you asked that first contestant to write the show a check for $200k he would laugh in your face, even though that’s essentially what he did. It’s funny how people consider found money less valuable than that which is earned.
I think you’re making the unreasonable assumption that most of the contestants know enough about math or probability or game theory (you only need a basic knowledge of any one of those three subjects to “solve” the game) to apply it to the game.
I don’t even watch this show, because I know I’d be frustrated. When the show first started airing, my friends and I discussed the expected value calculation briefly and pretty much agreed that no contestant was going to do that and none of us were going to waste time watching the contestants not do that.
Making the right choice in this game is a little more complicated than just calculating the average of the remaining values (the mathematical expectation). If the offer exceeds the expectation, then it is right to take it, but it is sometimes right to take a slightly low offer. If the two remaining amounts are a million and a penny, a poor person should probably take a $450,000 offer, but a rich person should probably take a chance on getting the million. For more on why this is the case, check out this essay.
I am surprised that you gamble. Texas Hold’em?
I would be a terrible Deal or No Deal contestant. I always assume that things are going to turn out well for me. I like to risk it all.
Back to Texas Hold’em – I am terrible at that too because I bluff every hand or assume that I am going to hit “runner, runner”.
i don’t gamble
This show is huge in the UK. I quite like predicting what the offers will be throughout the show, and also trying to guess whether they’re going to take it or not.
I think the behind the scenes set up where they are in a hotel together for days at a time, each hoping to get picked to go on by the producers contributes to the irrationality displayed. Which is fine, cos it’s great tv.
I’m a very conservative sort of person with money…I definitely would go for the bank offer.
I bet one of the initial screening questions for wannabe contestants is: Do you play the lottery?
BTW you’d have to fold in a utility function in the expectation integral as well.
I love to play poker and other card games, but those rely on skill as much as luck. With something like this, I am of the opinion that a bird in hand is better than two in the bush.
I gotta say that I would probably take any decent offer as soon as I could, as even just a few grand would make a difference in my life. At least more than winning nothing would! Great post, love the analysis.
@ Rocket: Nope, not poker. Just blackjack at the tables (card counting of course)!
I’ve always wanted to write down all the cases remaining as well as the banks offers to determine the formula – I’m such a nerd.
What I think is most ridiculous is that people are like “I had a dream that the $1M is in case 6, so I know it is in here” rather than realizing that it is all about probability.
Except Oprah would probably want to give away the money to someone, so her threshold would be lowered.